I had a pitiful week working on KA. I made it through one video, then began a second and was getting my ass kicked so badly that I stopped watching. And that’s where my week ended on KA… I feel like the math that’s being used in LA isn’t too difficult to understand – it’s just the fundamental operations of addition, subtraction, multiplication and division – but I’m having a hard time understanding how those operations are applied to vectors and matrices, and what the solutions represent. In the only video I watched all the way through on KA, a lot of what was taught had more to do with definitions and general concepts than understanding/practicing how to do the math behind it. This is what I’m having difficulty grasping. I find a lot of the notation very confusing. 😔 So, all in all, I got rocked on KA, BUT I made up for it a bit by branching out and watching a few videos from the YouTube creators Wrath of Math and Dr. Trefor Bazett. After watching their videos, I’ve now come up with a new game plan for next week which I’ll talk about at the end of this post.
As I said, the KA video I watched from start to finish – the seventh video from the section Null Space and Column Space – was very hard for me to understand. It was titled Visualizing a column space as a plane in R3 and it was a continuation from the sixth video from the series, which was the final video I watched last week – it showed how to find a “basis vectors” of a matrix, whatever that means. Nonetheless, here are three screen shots from the start of the video which show how to find the basis vectors of the given matrix:



As far as I understand it, what everything above boils down to is that the last two column vectors in the matrix are redundant as you can use some scaler combination of the first two vectors to equal the last two vectors. This means (I think) that the first two vectors could be considered the “basis vectors” of this particular matrix. I believe what this also means if you scale and add the first two vectors in infinite combinations, you can in theory create a plane. The last two vectors lay on that theoretical, infinite plan which is why they’re considered redundant in this matrix. (FYI, there’s a very good chance everything I just wrote is wrong.)
The next part of the video shows how to find a normal vector to the plane by stating that the cross product of the basis vectors – which would result in a normal vector – dotted with a vector on the plan would be equal to 0. (That could also be wrong.) It also shows that by subtracting a generalized vector, [x, y, z], from a vector on the plane before taking the dot product, you’re left with the equation for the plan created by the basis vectors. (Again, I’m likely wrong.) Here’s a screen shot of what I’m talking about and my notes:


I’m not going to try and explain what the final part of the video was talking about, because I have no clue. The one thing I took away from the final part of the video was that I sort of started to understand how/why turning matrices into row echelon form can be useful to solve things like finding the equation of a plane. The math and the notation went way over my head, but it was nice to see that this is all leading somewhere. Here’s a screen shot from that final part of the vid and the notes I copied from it:



After grinding my way through that video, I started watching the eighth vid, Proof: Any Subspace Basis has Same Number of Elements, and got rocked so hard I gave up watching it after four minutes. I decided to Google “Basis Vectors” to try to get a better understanding of what they are and one of the first videos that popped up was a Wrath of Math vid. That video definitely gave me a better understanding of what basis vectors are, but I still don’t completely understand how or why the math works. I’m not going to explain my notes, but here are some screen shots from that vid and the notes I took:




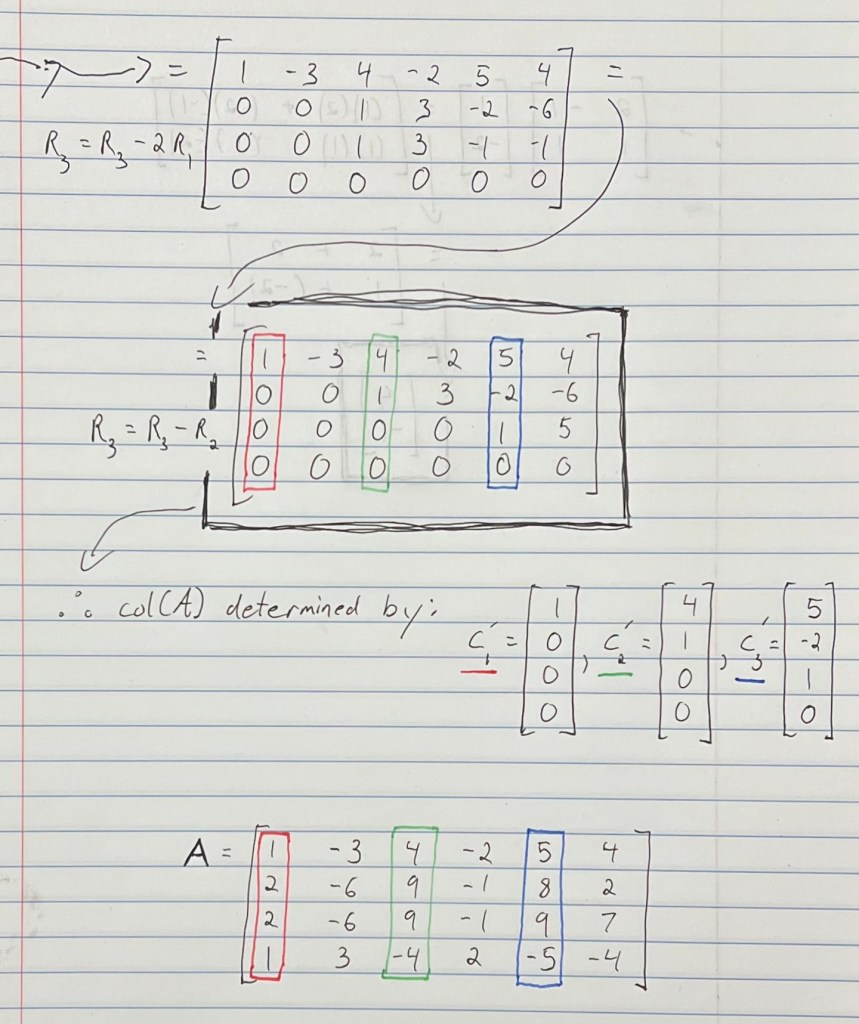
(In my last note above, where it says C1’ = […], C2’ = […], and C3’ = […], instead of it being C2’ and C3’ it should be C3’ and C5’ to denote the third and fifth column vectors in matrix A, along with the first column vector.)
By the end of the week, I started thinking I need to do a hard reset on Linear Algebra. I Googled “Linear Algebra” hoping to find a playlist of vids to start watching from the start and came across the following video. I was happy to find out it is the first video in an 82-video long playlist titled Linear Algebra (Full Course):
I’ve seen videos from this creator, Dr. Trefor Bazett, before. I found this intro video SUPER helpful for me to understand the general idea of what’s going on with linear algebra. The video definitely helped to simplify some things in my head. For instance, the idea that the green vector in this video can be rewritten using the red and yellow unit vectors was very helpful, especially the way he graphed it. It also helped me get a stronger intuitive grasp on why matrix multiplication works and looks the way it does. Here are some screen shots and my notes from that part of the video:





The last few KA videos I’ve watched have all been >20 minutes long and I’ve found them very difficult to follow along with them. The videos in this Linear Algebra playlist by Trefor are all 3-11 minutes long, most of them being around six or seven minutes. At least this coming week, my game plan is to switch from KA to that playlist by Trefor to see if I can get a better grasp on linear algebra, in general, before going back to the KA vids. I’m disappointed about doing this since it means that it’ll take me ever longer to finish KA, but I think in the big picture it will end up making things a lot easier. That’s the hope at least! 🤞🏼
It’s hard to believe that this is my FINAL week of my FIFTH year working on KA… 😳 Thinking back to when I started this in September of 2019, it’s hard to believe that this endeavor has become such a huge part of my life and (not to be too deep, but…) a large part of my identity. I like that I can point to this blog as evidence that I’m doing things to improve myself, even if I don’t really know where it’s all leading. Although I’m bummed that learning calculus has taken me WAY longer than I wanted/expected it to, I know I’m making progress and will get there eventually and, more importantly, I’m making progress towards becoming a better version of myself. 💪🏼